Page 42 - 2024-bfw-starnes-TPS7e-SE proofs.indd

SecTIoN 1c Displaying Quantitative Data with Graphs 29

Notice that in the preceding example, we discussed the distributions of house-
hold size only for the two samples of 50 households. We might be interested in
whether the sample data give us convincing evidence of a difference in the popu-
lation distributions of household size for South Africa and the United Kingdom.
We’ll have to wait several units to decide whether we can reach such a conclu-
sion, but our ability to make such an inference later will be helped by the fact
© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only - Do Not Copy
that the households in our samples were chosen at random.

Displaying Quantitative Data: Stemplots

Another simple type of graph for displaying quantitative data is a stemplot, also
called a stem-and-leaf plot.

DEFINITION Stemplot
A stemplot shows each data value separated into two parts: a stem, which consists
of the leftmost digits, and a leaf, consisting of the final digit. The stems are ordered
from least to greatest and arranged in a vertical column. The leaves are arranged in
increasing order out from the appropriate stems.

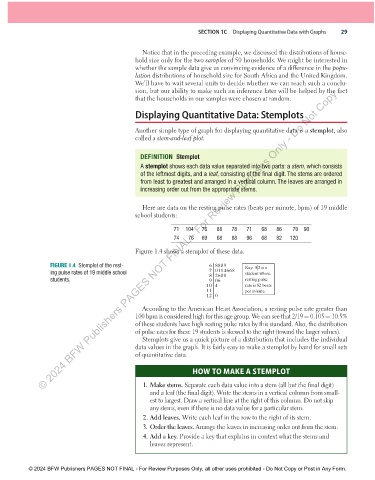
Here are data on the resting pulse rates (beats per minute, bpm) of 19 middle
school students:

71 104 76 88 78 71 68 86 70 90
74 76 69 68 88 96 68 82 120

Figure 1.4 shows a stemplot of these data.

FIGURE 1.4 Stemplot of the rest- 6 8889 Key: 8|2 is a
ing pulse rates of 19 middle school 7 0114668 student whose
8 2688
students. 9 06 resting pulse
10 4 rate is 82 beats
11 per minute.
12 0
According to the American Heart Association, a resting pulse rate greater than
100 bpm is considered high for this age group. We can see that 2/19 = 0.105 = 10.5%
of these students have high resting pulse rates by this standard. Also, the distribution
of pulse rates for these 19 students is skewed to the right (toward the larger values).
Stemplots give us a quick picture of a distribution that includes the individual
data values in the graph. It is fairly easy to make a stemplot by hand for small sets
of quantitative data.

HOW TO MAKE A STEMPLOT

1. Make stems. Separate each data value into a stem (all but the final digit)
and a leaf (the final digit). Write the stems in a vertical column from small-
est to largest. Draw a vertical line at the right of this column. Do not skip
any stems, even if there is no data value for a particular stem.
2. Add leaves. Write each leaf in the row to the right of its stem.
3. Order the leaves. Arrange the leaves in increasing order out from the stem.
4. Add a key. Provide a key that explains in context what the stems and
leaves represent.

© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only, all other uses prohibited - Do Not Copy or Post in Any Form.

02_StarnesTPS7e_40934_un01_p1_001_086_6pp.indd 29 13/09/23 5:37 PM