Page 76 - 2024-bfw-starnes-TPS7e-SE proofs.indd

SecTIoN 1D Describing Quantitative Data with Numbers 63

CHECK YOUR
UNDERSTANDING

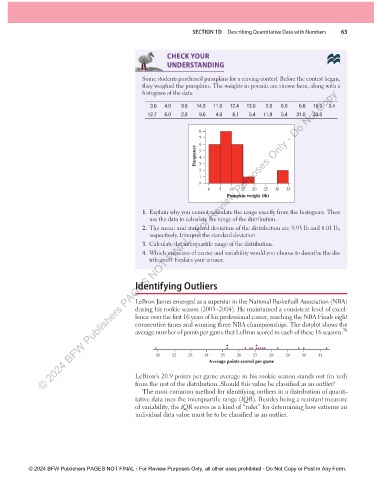
Some students purchased pumpkins for a carving contest. Before the contest began,
they weighed the pumpkins. The weights in pounds are shown here, along with a
© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only - Do Not Copy
histogram of the data.
3.6 4.0 9.6 14.0 11.0 12.4 13.0 2.0 6.0 6.6 15.0 3.4
12.7 6.0 2.8 9.6 4.0 6.1 5.4 11.9 5.4 31.0 33.0

8
7
Frequency 6 5 4

2 3
1
0
0 5 10 15 20 25 30 35
Pumpkin weight (lb)

1. Explain why you cannot calculate the range exactly from the histogram. Then

use the data to calculate the range of the distribution.

2. The mean and standard deviation of the distribution are 9.93 lb and 8.01 lb,
respectively. Interpret the standard deviation.

3. Calculate the interquartile range of the distribution.

4. Which measures of center and variability would you choose to describe the dis-
tribution? Explain your answer.

Identifying outliers

LeBron James emerged as a superstar in the National Basketball Association (NBA)
during his rookie season (2003–2004). He maintained a consistent level of excel-
lence over the first 16 years of his professional career, reaching the NBA Finals eight
consecutive times and winning three NBA championships. The dotplot shows the
average number of points per game that LeBron scored in each of these 16 seasons. 70

21 22 23 24 25 26 27 28 29 30 31
Average points scored per game

LeBron’s 20.9 points per game average in his rookie season stands out (in red)
from the rest of the distribution. Should this value be classified as an outlier?
The most common method for identifying outliers in a distribution of quanti-
tative data uses the interquartile range ( IQR ). Besides being a resistant measure
of variability, the IQR serves as a kind of “ruler” for determining how extreme an
individual data value must be to be classified as an outlier.

© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only, all other uses prohibited - Do Not Copy or Post in Any Form.

02_StarnesTPS7e_40934_un01_p1_001_086_6pp.indd 63 13/09/23 5:39 PM