Page 71 - 2024-bfw-starnes-TPS7e-SE proofs.indd

58 UNIT 1 Exploring One-Variable Data

PROPERTIES OF THE STANDARD DEVIATION
More important than the details of calculating s are the properties of the stan-
x
dard deviation as a measure of variability:
• s is always greater than or equal to 0. s x = 0 only when there is no
x
variability — that is, when all values in a distribution are the same.
© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only - Do Not Copy
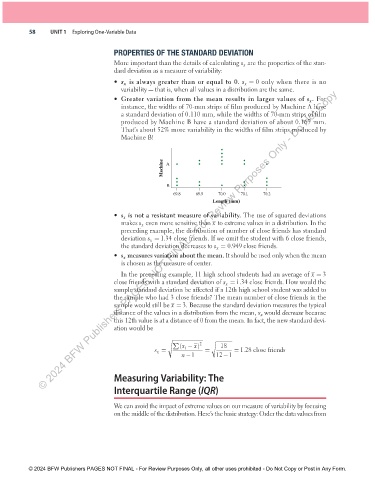
• Greater variation from the mean results in larger values of s . For
x
instance, the widths of 70-mm strips of film produced by Machine A have
a standard deviation of 0.110 mm, while the widths of 70-mm strips of film
produced by Machine B have a standard deviation of about 0.167 mm.
That’s about 52% more variability in the widths of film strips produced by
Machine B!

Machine A

B
69.8 69.9 70.0 70.1 70.2
Length (mm)

• s is not a resistant measure of variability. The use of squared deviations
x
makes s even more sensitive than x to extreme values in a distribution. In the
x
preceding example, the distribution of number of close friends has standard
deviation s x = 1.34 close friends. If we omit the student with 6 close friends,
the standard deviation decreases to s x = 0.949 close friends.
• s measures variation about the mean. It should be used only when the mean
x
is chosen as the measure of center.
In the preceding example, 11 high school students had an average of = 3x
close friends with a standard deviation of s x = 1.34 close friends. How would the
sample standard deviation be affected if a 12th high school student was added to
the sample who had 3 close friends? The mean number of close friends in the
sample would still be = 3x . Because the standard deviation measures the typical
distance of the values in a distribution from the mean, s would decrease because
x
this 12th value is at a distance of 0 from the mean. In fact, the new standard devi-
ation would be

Σ (x − ) x 2 18
s x = i = = 1.28 closefriends
n −1 12 −1

Measuring Variability: The

Interquartile Range (IQR)

We can avoid the impact of extreme values on our measure of variability by focusing
on the middle of the distribution. Here’s the basic strategy: Order the data values from

© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only, all other uses prohibited - Do Not Copy or Post in Any Form.

02_StarnesTPS7e_40934_un01_p1_001_086_6pp.indd 58 13/09/23 5:39 PM