Page 70 - 2024-bfw-starnes-TPS7e-SE proofs.indd

SecTIoN 1D Describing Quantitative Data with Numbers 57

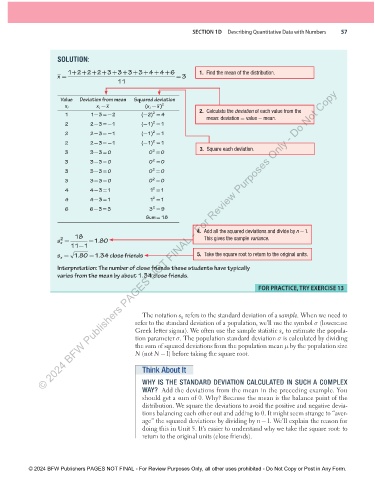
SOLUTION:

++++
+++
1 222 3333 4+++ 1. Find the mean of the distribution.
4 6
x = = 3
11
© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only - Do Not Copy
Value Deviation from mean Squared deviation
x i − x (x i − ) x 2
x i 2. Calculate the deviation of each value from the
2
−
1 13−=− 2 ( 2) = 4
mean: deviation = value − mean.
2
−
2 2 3−=− 1 ( 1) = 1
2
−
2 2 3−=− 1 ( 1) = 1
2
−
2 2 3−=− 1 ( 1) = 1
2
3 33−= 0 0 = 0 3. Square each deviation.
2
3 33−= 0 0 = 0
2
3 33−= 0 0 = 0
2
3 33−= 0 0 = 0
2
31
4 4−= 1 = 1
2
31
4 4−= 1 = 1
2
6 6 3−= 3 3 = 9
Sum = 18
4. Add all the squared deviations and divide by −1.
n
18 This gives the sample variance.
s 2 = = 1.80
x
−
11 1
s x = 1.80 = 1.34 closefriends 5. Take the square root to return to the original units.
Interpretation: The number of close friends these students have typically
varies from the mean by about 1.34 close friends.
FoR PRAcTIce, TRY eXeRcISe 13
The notation s refers to the standard deviation of a sample. When we need to
x
refer to the standard deviation of a population, we’ll use the symbol σ (lowercase
Greek letter sigma). We often use the sample statistic s to estimate the popula-
x
tion parameter σ. The population standard deviation σ is calculated by dividing
the sum of squared deviations from the population mean µ by the population size
N (not −1N ) before taking the square root.

Think About It

WHY IS THE STANDARD DEVIATION CALCULATED IN SUCH A COMPLEX
WAY? Add the deviations from the mean in the preceding example. You
should get a sum of 0. Why? Because the mean is the balance point of the
distribution. We square the deviations to avoid the positive and negative devia-
tions balancing each other out and adding to 0. It might seem strange to “aver-
age” the squared deviations by dividing by −1n . We’ll explain the reason for
doing this in Unit 5. It’s easier to understand why we take the square root: to
return to the original units (close friends).

© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only, all other uses prohibited - Do Not Copy or Post in Any Form.

02_StarnesTPS7e_40934_un01_p1_001_086_6pp.indd 57 13/09/23 5:39 PM