Page 65 - 2024-bfw-starnes-TPS7e-SE proofs.indd

52 UNIT 1 Exploring One-Variable Data

PROPERTIES OF THE MEAN
The preceding example illustrates an important weakness of the mean as a mea-
sure of center: The mean is not resistant to extreme values, such as outliers. The
bag of Fritos, with only 19% air, decreases the mean by 1.81 percentage points.

© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only - Do Not Copy
DEFINITION Resistant
A statistical measure is resistant if it is not affected much by extreme data values.

The median is a resistant measure of center. In the preceding example, the
median percent air in all 14 bags of chips is 45.5. If we remove the possible outlier
bag of Fritos, the median percent air in the remaining 13 bags is nearly the same (46).
Why is the mean so sensitive to extreme values? The following activity pro-
vides some insight.

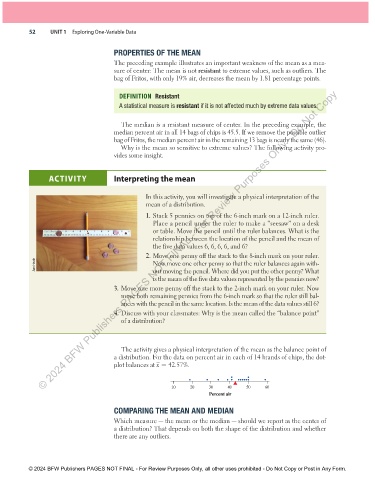
ACTIVITY Interpreting the mean

In this activity, you will investigate a physical interpretation of the
mean of a distribution.
1. Stack 5 pennies on top of the 6-inch mark on a 12-inch ruler.
Place a pencil under the ruler to make a “seesaw” on a desk
or table. Move the pencil until the ruler balances. What is the
relationship between the location of the pencil and the mean of
the five data values 6, 6, 6, 6, and 6?
2. Move one penny off the stack to the 8-inch mark on your ruler.
Ann Heath Now move one other penny so that the ruler balances again with-
out moving the pencil. Where did you put the other penny? What
is the mean of the five data values represented by the pennies now?
3. Move one more penny off the stack to the 2-inch mark on your ruler. Now
move both remaining pennies from the 6-inch mark so that the ruler still bal-
ances with the pencil in the same location. Is the mean of the data values still 6?
4. Discuss with your classmates: Why is the mean called the “balance point”
of a distribution?

The activity gives a physical interpretation of the mean as the balance point of
a distribution. For the data on percent air in each of 14 brands of chips, the dot-
plot balances at x = 42.57%.

10 20 30 40 50 60
Percent air

COMPARING THE MEAN AND MEDIAN

Which measure — the mean or the median — should we report as the center of
a distribution? That depends on both the shape of the distribution and whether
there are any outliers.

© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only, all other uses prohibited - Do Not Copy or Post in Any Form.

02_StarnesTPS7e_40934_un01_p1_001_086_6pp.indd 52 13/09/23 5:38 PM