Page 64 - 2024-bfw-starnes-TPS7e-SE proofs.indd

SecTIoN 1D Describing Quantitative Data with Numbers 51

EXAMPLE More chips please Skill 2.C
Measuring center: The mean

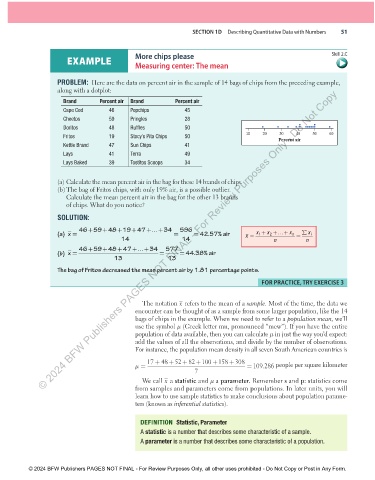
PROBLEM: Here are the data on percent air in the sample of 14 bags of chips from the preceding example,
along with a dotplot:
© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only - Do Not Copy
Brand Percent air Brand Percent air
Cape Cod 46 Popchips 45
Cheetos 59 Pringles 28
Doritos 48 Ruffles 50
Fritos 19 Stacy’s Pita Chips 50 10 20 30 40 50 60
Percent air
Kettle Brand 47 Sun Chips 41
Lays 41 Terra 49
Lays Baked 39 Tostitos Scoops 34

(a) Calculate the mean percent air in the bag for these 14 brands of chips.
(b) The bag of Fritos chips, with only 19% air, is a possible outlier.

Calculate the mean percent air in the bag for the other 13 brands
of chips. What do you notice?

SOLUTION:
+
+
46 59 48 19 47 …+ 34 596
+
+
+
(a) x = = = 42.57% air x = x 1 + x 2 +… + x n = ∑ x i
14 14 n n
+
+
+
46 59 48 47 …+ 34 577
+
(b) x = = = 44.38% air
13 13
The bag of Fritos decreased the mean percent air by 1.81 percentage points.
FoR PRAcTIce, TRY eXeRcISe 3
The notation x refers to the mean of a sample. Most of the time, the data we

encounter can be thought of as a sample from some larger population, like the 14
bags of chips in the example. When we need to refer to a population mean, we’ll

use the symbol µ (Greek letter mu, pronounced “mew”). If you have the entire

population of data available, then you can calculate µ in just the way you’d expect:

add the values of all the observations, and divide by the number of observations.
For instance, the population mean density in all seven South American countries is
17 + 48 + 52 + 82 + 100 + 158 + 308
µ = = 109.286 people per square kilometer
7

We call x a statistic and µ a parameter. Remember s and p: s tatistics come

from s amples and p arameters come from p opulations. In later units, you will
learn how to use sample statistics to make conclusions about population parame-
ters (known as inferential statistics ).
DEFINITION Statistic, Parameter
A statistic is a number that describes some characteristic of a sample.
A parameter is a number that describes some characteristic of a population.

© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only, all other uses prohibited - Do Not Copy or Post in Any Form.

02_StarnesTPS7e_40934_un01_p1_001_086_6pp.indd 51 13/09/23 5:38 PM