Page 74 - 2024-bfw-starnes-TPS7e-SE proofs.indd
P. 74
SecTIoN 1D Describing Quantitative Data with Numbers 61
and whether there are any outliers. As you learned earlier, the median is a resis-
tant measure of center but the mean is not. Among measures of variability, the
interquartile range ( IQR ) is resistant to extreme values but the range and stan-
dard deviation are not. For now, follow this advice:
• If a distribution of quantitative data is roughly symmetric with no outliers, the
© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only - Do Not Copy
mean x and standard deviation s are the preferred measures of center and
x
variability.
• If the distribution is clearly skewed or has outliers, use the median as the mea-
sure of center and the IQR as the measure of variability.
CHOOSING MEASURES OF CENTER AND VARIABILITY
The median and IQR are usually better choices than the mean and standard
deviation for describing a skewed distribution or a distribution with outliers.
Use x and s for roughly symmetric distributions that don’t have outliers.
x
We recommend using the range to measure variability only as a last resort because
it gives so little information about how the individual data values are distributed.
EXAMPLE Lead in the water Skill 4.B
choosing summary statistics
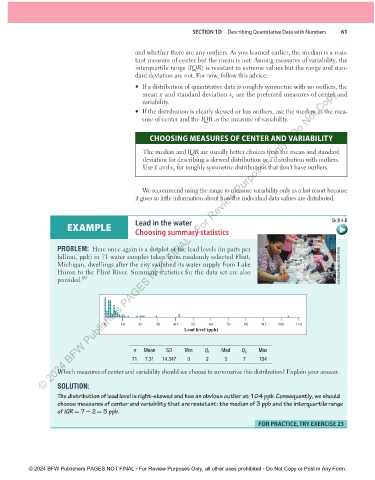
PROBLEM: Here once again is a dotplot of the lead levels (in parts per
billion, ppb) in 71 water samples taken from randomly selected Flint,
Michigan, dwellings after the city switched its water supply from Lake Jim West/Alamy Stock Photo
Huron to the Flint River. Summary statistics for the data set are also
provided. 69
0 10 20 30 40 50 60 70 80 90 100 110
Lead level (ppb)
n Mean SD Min Q 1 Med Q 3 Max
71 7.31 14.347 0 2 3 7 104
Which measures of center and variability should we choose to summarize this distribution? Explain your answer.
SOLUTION:
The distribution of lead level is right-skewed and has an obvious outlier at 104 ppb. Consequently, we should
choose measures of center and variability that are resistant: the median of 3 ppb and the interquartile range
of IQR = 7 − 2 = 5 ppb.
FoR PRAcTIce, TRY eXeRcISe 23
© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only, all other uses prohibited - Do Not Copy or Post in Any Form.
02_StarnesTPS7e_40934_un01_p1_001_086_6pp.indd 61 13/09/23 5:39 PM