Page 24 - 2024-bfw-starnes-TPS7e-SE proofs.indd

SecTIoN 1B Displaying and Describing Categorical Data 11

common way to display categorical data is with a bar graph (also known as a bar
chart).

DEFINITION Bar graph
A bar graph shows each category as a bar. The heights of the bars show the cate-
© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only - Do Not Copy
gory frequencies or relative frequencies.

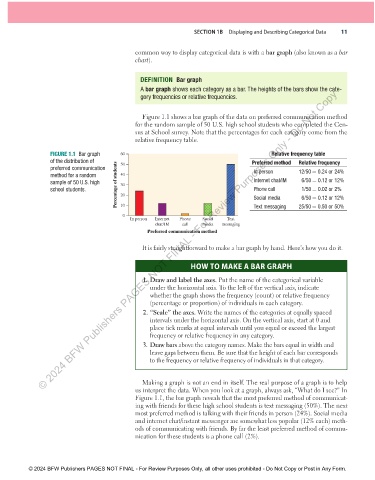
Figure 1.1 shows a bar graph of the data on preferred communication method
for the random sample of 50 U.S. high school students who completed the Cen-
sus at School survey. Note that the percentages for each category come from the
relative frequency table.

FIGURE 1.1 Bar graph 60 Relative frequency table
of the distribution of 50 Preferred method Relative frequency
preferred communication In person 12/50 = 0.24 or 24%
method for a random 40
sample of 50 U.S. high Percentage of students 30 Internet chat/IM 6/50 = 0.12 or 12%
school students. 20 Phone call 1/50 = 0.02 or 2%
6/50 = 0.12 or 12%
Social media
10
0 Text messaging 25/50 = 0.50 or 50%
In person Internet Phone Social Text
chat/IM call media messaging
Preferred communication method

It is fairly straightforward to make a bar graph by hand. Here’s how you do it.

HOW TO MAKE A BAR GRAPH

1. Draw and label the axes. Put the name of the categorical variable
under the horizontal axis. To the left of the vertical axis, indicate
whether the graph shows the frequency (count) or relative frequency
(percentage or proportion) of individuals in each category.
2. “Scale” the axes. Write the names of the categories at equally spaced
intervals under the horizontal axis. On the vertical axis, start at 0 and
place tick marks at equal intervals until you equal or exceed the largest
frequency or relative frequency in any category.
3. Draw bars above the category names. Make the bars equal in width and
leave gaps between them. Be sure that the height of each bar corresponds
to the frequency or relative frequency of individuals in that category.

Making a graph is not an end in itself. The real purpose of a graph is to help
us interpret the data. When you look at a graph, always ask, “What do I see?” In
Figure 1.1, the bar graph reveals that the most preferred method of communicat-
ing with friends for these high school students is text messaging (50%). The next
most preferred method is talking with their friends in person (24%). Social media
and internet chat/instant messenger are somewhat less popular (12% each) meth-
ods of communicating with friends. By far the least preferred method of commu-
nication for these students is a phone call (2%).

© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only, all other uses prohibited - Do Not Copy or Post in Any Form.

02_StarnesTPS7e_40934_un01_p1_001_086_6pp.indd 11 13/09/23 5:36 PM