Page 70 - 2024-calc4e-SE proofs-4e.indd

Sullivan 04 apcalc4e 45342 ch02 166 233 5pp August 7, 2023 12:54

220 Chapter 2 • The Derivative and Its Properties

EXAMPLE 2 Differentiating Trigonometric Functions
CALC CLIP
Find the derivative of each function:
cos θ e t
2
(a) f (x) = x cos x (b) g(θ) = (c) F(t) =
1 − sin θ cos t
Solution
© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only - Do Not Copy.
(a) ′ d 2 2 d d 2
f (x) = (x cos x) = x cos x + x (cos x)
dx dx dx
2
2
= x (−sin x) + 2x cos x = 2x cos x − x sin x

d d
cos θ (1 − sin θ) − (cos θ) (1 − sin θ)

(b) d cos θ dθ dθ
′
g (θ) = =
dθ 1 − sin θ (1 − sin θ) 2
2
2
−sin θ (1 − sin θ) − cos θ(−cos θ) −sin θ + sin θ + cos θ
= 2 = 2
(1 − sin θ) (1 − sin θ)
−sin θ + 1 1
= 2 =
(1 − sin θ) 1 − sin θ

d t t d
e (cos t) − e cos t
t
t
d e t dt dt e cos t − e (−sin t)

′
(c) F (t) = = =
2
2
dt cos t cos t cos t
t
e (cos t + sin t)
= 2
cos t
R
NOW WORK Problem 13 and AP Practice Problems 2, 6, and 8.
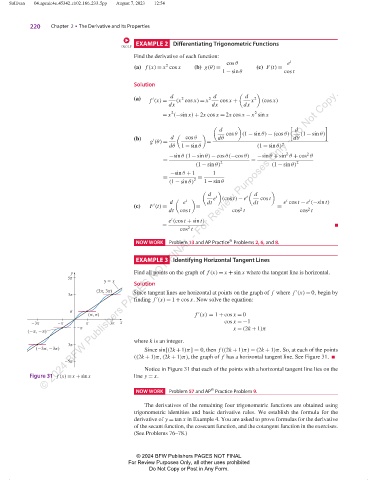
EXAMPLE 3 Identifying Horizontal Tangent Lines
y Find all points on the graph of f (x) = x + sin x where the tangent line is horizontal.
5π
y x
Solution
(3π, 3π) Since tangent lines are horizontal at points on the graph of f where f (x) = 0, begin by
′
3π
finding f (x) = 1 + cos x. Now solve the equation:
′
π
′
(π, π) f (x) = 1 + cos x = 0
x cos x = −1
3π π π 3π
π x = (2k + 1)π
(π, π)
where k is an integer.
3π
(3π, 3π) Since sin[(2k +1)π] = 0, then f ((2k + 1)π) = (2k + 1)π. So, at each of the points
((2k + 1)π, (2k + 1)π), the graph of f has a horizontal tangent line. See Figure 31.
5π
Notice in Figure 31 that each of the points with a horizontal tangent line lies on the
Figure 31 f (x) = x + sin x line y = x.
R
NOW WORK Problem 57 and AP Practice Problem 9.
The derivatives of the remaining four trigonometric functions are obtained using
trigonometric identities and basic derivative rules. We establish the formula for the
derivative of y = tan x in Example 4. You are asked to prove formulas for the derivative
of the secant function, the cosecant function, and the cotangent function in the exercises.
(See Problems 76–78.)

© 2024 BFW Publishers PAGES NOT FINAL
For Review Purposes Only, all other uses prohibited
Do Not Copy or Post in Any Form.