Page 33 - 2024-calc4e-SE proofs-4e.indd

Sullivan 04 apcalc4e 45342 ch02 166 233 5pp August 7, 2023 12:54

Section 2.2 • The Derivative as a Function; Differentiability 183

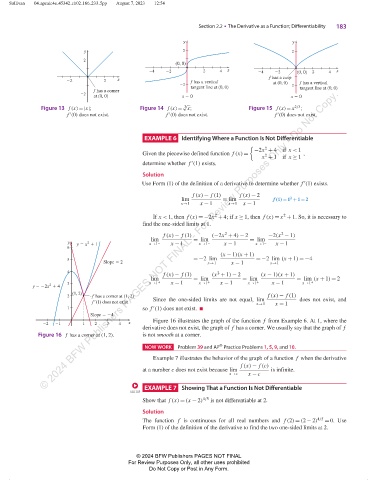
y y
y 2 2
2
(0, 0)
4 2 2 4 x 24 22 (0, 0) 2 4 x
f has a cusp
2 2 x f has a vertical
2 at (0, 0) 22 f has a vertical
© 2024 BFW Publishers PAGES NOT FINAL - For Review Purposes Only - Do Not Copy.
tangent line at (0, 0) tangent line at (0, 0)
f has a corner
2
at (0, 0) x 0 x 5 0
√ 2/3
3
Figure 13 f (x) = |x|; Figure 14 f (x) = x; Figure 15 f (x) = x ;
f (0) does not exist. f (0) does not exist. f (0) does not exist.
′
′
′
EXAMPLE 6 Identifying Where a Function Is Not Differentiable
2
−2x + 4 if x < 1
Given the piecewise defined function f (x) = 2 ,
x + 1 if x ≥ 1
determine whether f (1) exists.
′
Solution
Use Form (1) of the definition of a derivative to determine whether f (1) exists.
′
f (x) − f (1) f (x) − 2
2
lim = lim f (1) = 1 + 1 = 2
x→1 x − 1 x→1 x − 1
2
2
If x < 1, then f (x) = −2x + 4; if x ≥ 1, then f (x) = x + 1. So, it is necessary to
find the one-sided limits at 1.
2
2
f (x) − f (1) (−2x + 4) − 2 −2(x − 1)
lim = lim = lim
y 2
y x 1 x→1 − x − 1 x→1 − x − 1 x→1 − x − 1
6
(x − 1)(x + 1)
5 = −2 lim = −2 lim (x + 1) = −4
Slope 2 x→1 − x − 1 x→1 −
4 2
f (x) − f (1) (x + 1) − 2 (x − 1)(x + 1)
lim = lim = lim = lim (x + 1) = 2
3 x→1 + x − 1 x→1 + x − 1 x→1 + x − 1 x→1 +
2
y 2x 4
(1, 2)
2 f has a corner at (1, 2) f (x) − f (1)
f (1) does not exist Since the one-sided limits are not equal, lim does not exist, and
x→1 x − 1
1 so f (1) does not exist.
′
Slope 4
Figure 16 illustrates the graph of the function f from Example 6. At 1, where the
2 1 1 2 3 4 x
derivative does not exist, the graph of f has a corner. We usually say that the graph of f
Figure 16 f has a corner at (1, 2). is not smooth at a corner.
R
NOW WORK Problem 39 and AP Practice Problems 1, 5, 9, and 10.
Example 7 illustrates the behavior of the graph of a function f when the derivative
f (x) − f (c)
at a number c does not exist because lim is infinite.
x→c x − c
EXAMPLE 7 Showing That a Function Is Not Differentiable
CALC CLIP
Show that f (x) = (x − 2) 4/5 is not differentiable at 2.
Solution
The function f is continuous for all real numbers and f (2) = (2 − 2) 4/5 = 0. Use
Form (1) of the definition of the derivative to find the two one-sided limits at 2.

© 2024 BFW Publishers PAGES NOT FINAL
For Review Purposes Only, all other uses prohibited
Do Not Copy or Post in Any Form.